Really Freakin' Interesting Device

The University of Michigan is on break right now, which means I need my ID card to get into my office building.  It got me thinking about how the system is reading my card – Originally, the cards used a magnetic stripe, but a few years ago they swapped those for RFID, so I thought I'd look into how each of those works.

The magnetic stripe consists of a series of zones, where the field points up or down, corresponding to 1 or 0:

From Wikipedia

This is the same way hard drives store data.  A read/write head moves over the zones sensing and changing their field as needed.  This is why getting a powerful magnet near your card can scramble the data.  The stripe begins with a start pattern that tells the reader how fast the card is moving, so changing speed mid-swipe can also cause an error.

RFID tags use radio-waves, which can be sensed from a distance.  The trouble is, producing radio-waves requires power, and it would be inconvenient to attach a battery to every card.  That's where RFID tags get clever:

From Priority 1 Design

The card reader, which is attached to a power source, sends out a wave that gets absorbed by the RFID tag.  That wave carries enough energy to power a microchip that modulates how much is absorbed.  The reader then senses how much of the power it sent out is lost over time, giving the series of 1s and 0s needed to transmit information.

When I was growing up, and dreaming of being a scientist, one of my planned inventions (aside from my mother's "Beam me up" technology) was wireless power.  It seems I'm about 70 years late for that, but there's plenty more to discover!

Momentous Holidays

This week's question comes from my nephew Ezra:


How come I have to push my arm out to move?

What you're experiencing is conservation of angular momentum.  Regular linear momentum is given by the mass times the velocity

This is why fast, heavy things are harder to catch than slow, light things – You have to overcome their momentum to make them stop.  Similarly, if you want to get something moving really fast, it pushes back on you to cancel out the momentum you impart to it.  Hence the recoil in firing a gun.

Angular momentum is the same idea applied to things moving in a circle.  The classic example is a figure skater spinning at different speeds, which I've mentioned before, but it applies here too.  When you push your arm out, part of that motion is going around the seat, so it has angular momentum.  That gets transferred to the seat, making it turn in the opposite direction.

Here are two experiments you can try: What happens if you hold your arm out and pull in, instead of pushing outward?  What happens when you push your arm towards the center of the seat, instead of to the side?

A Long Haul

 

We've already had inches of snow building up in Ann Arbor, making it a little more difficult to get around, and it got me wondering about snow removal in a city.  For large snowfalls, there isn't really room for big piles, so the snow would need to be hauled elsewhere.  How much cheaper is that than melting the snow with, say, blowtorches?

Melting snow involves overcoming the latent heat of the ice crystals.  This is the energy contained in the crystalline structure – I've mentioned this property before in the case of candle wax.  If we assume the snow is already at the melting point of 0°C, then we still need 334 kJ of energy per kilogram of snow.

Figuring out the energy needed to move the snow is a little more complicated, since it involves converting the gas mileage.  Current semi trucks get about 6.5 mpg with diesel fuel.  Diesel engines are relatively efficient (though they produce nastier byproducts), converting about 45% of the chemical energy into mechanical work.  The final piece we need is the amount of energy in a given volume of fuel, 35.8 MJ/L.  Putting these together, we can find the energy needed to move a truckload of snow a given distance: 2.16 x 10^-5 miles/kJ.

The maximum weight this truck can carry is 80,000 pounds, so we can calculate the energy to melt that much, 1.21 x 10^10 Joules (not jigawatts, sadly).  So how far would we need to haul the snow to make it more efficient to melt?  26 million miles, or several trips to the moon and back, but only 1/5th of the way to Mars.

I wasn't expecting to have a breakthrough snow removal technique, but this does seem a little high.  It's possible I'll find a mistake later, so take these results with a grain of salt (yet another snow-removal method).

Correction: I left out a factor of 10^-5 in my calculation above.  The actual distance is 262 miles.  Thanks, Kevin!

Not with a Bang

Another question this week from my grandfather-in-law-to-be, George: How does entropy work on the scale of the universe? Doesn't gravity reduce entropy by pulling things together?

Before I get into this, I should first explain what entropy is.  I've mentioned it before as a type of randomness, but it's a bit more complicated.  First, there's the idea of microstates and macrostates.  Imagine we have a box with 10 particles bouncing around inside, and we take a photo at two different times:

 These look basically the same, even though the particles are in different places.  These are two microstates belonging to the same macrostate.  Now we take another photo, and find this:

This is qualitatively different, and is therefore a different macrostate.  However, there are far fewer ways to put all the particles in one corner like that, so the number of microstates is much smaller.  Thermodynamics assumes that all microstates are equally likely, which is why we usually only see the macrostates that have many of them.

Entropy is a way of measuring how many microstates a configuration has.  It's defined as

where k_B is Boltzmann's constant, and Ω is the number of microstates.  The second law of thermodynamics says that entropy always increases in any process – In a way, it defines the progress of time.  A common example is a box with two gasses separated by a divider.  If you remove the divider, the gasses will mix, and there are astronomically more ways for them to be mixed than separated, so they will not return to the original state.

So now, back to the original question.  It seems as if increasing entropy would imply the universe would smooth out to an equal amount of matter everywhere, but gravity acts against that.  The key is that when gravity brings lots of matter together, you can end up with a black hole.  It is believed that black holes carry entropy proportional to the area of their event horizons, which in turn is proportional to the mass that has fallen into them.  Therefore by collecting matter, a black hole is not violating the second law.

That leaves us with a rather sad conclusion called the heat-death of the universe.  If entropy continues to increase, it will reach some maximum value, at which point the universe will be a uniform temperature at all points.  Differences in temperature are required to extract energy from a system, so heat-death implies that no processes can occur after that point.

This is the way the world ends
This is the way the world ends
This is the way the world ends
Not with a bang but a whimper.
        –T. S. Eliot

Resounding Success

Earlier this week, I was getting ready to do some baking, but noticed something strange when I set out my mixing bowl:

 

There appears to be some vibration in my apartment that the fork/bowl were picking up.  I shared the video on Facebook, and joked that perhaps a passing gravitational wave was responsible, but when I heard my nephew was interested, I decided to go a little more in-depth.

The reason the fork begins to rattle is that the vibration is near the fork's resonant frequency.  This is the frequency at which a system is best able to absorb energy.  This may sound complicated, but you already have experience with finding a resonant frequency if you've ever played on a swing-set.  The way you pump your legs, pushing forward and pulling pack, is exactly the type of motion I'm talking about.

As a physics student, I've had many opportunities to experiment with resonant frequencies in labs, and get a feel for how the different parameters interact.  I wasn't sure how helpful it would be to show you a few isolated examples, and I wondered how difficult it would be to design an interactive experiment in HTML5.  Thanks to the examples by Daniel Schroeder (who coincidentally wrote the thermal physics textbook I used a few years ago), I was able to put together a simple demo in a matter of hours!

Below, you'll find an animated pendulum with some sliders.  The pendulum has 3 forces acting on it: gravity, which is always pulling it toward its lowest point; a damping force, which slows the pendulum according to its current speed; and a sinusoidal driving force, which pushes and pulls the pendulum with a specific amplitude and frequency (think of the leg-pumping on the swing).

  Canvas not supported; please update your browser.

Damping

Driving Amp

Driving Freq

The units are set up such that the pendulum's natural frequency is 1.  With no damping, this is the same as the resonant frequency, but adding damping brings the resonant frequency down.  I found (0.2, 0.3, 0.9) to be a good set, but the whole point is to play around a bit.  Be sure to leave a comment if you find a particularly interesting setting!

I was amazed how simple it was to make this in HTML5, so I may do more of these in the future.  If you'd like to take a look, I've put a stand-alone version here, so you don't need to go digging through the page source.

Ising the Body Electric

Last week I was in Chicago, visiting my friend Kevin, so I didn't get around to putting up a post, but I did get some material for this week: I've been going to a lot of shows, concerts, and other group events lately, and it got me thinking about crowd dynamics, specifically standing ovations.  I figured there were two factors that determined whether someone would stand up during applause:

• The quality of the performance
• Whether nearby people were standing

These two conditions reminded me of the Ising model used to simulate magnetic materials.  The idea is that in a ferromagnetic material (like iron), we can imagine a bunch of arrows, called spins, that point up or down.  The energy of any particular spin is related to those of its neighbors: +1 for each opposite spin, and -1 for matching spin (remember that low-energy states are preferred).  Here's an example:

In the Ising model, we pick a random spin at each time step, and decide if it should be flipped.  The probability is given by the Boltzmann distribution,

where ΔE is the difference in energy between the current state and the flipped state, and kT is the thermal energy.  For cases where the new state is lower energy, we get P > 1, so a flip is guaranteed.  We can also introduce an overall field, which biases the system in one direction – This could represent a permanent magnet near the iron, for example.
So how does this relate to audiences?  Each person can represent a spin, and their neighbors influence their probability of standing or sitting.  Someone could stand independent of their neighbors for an exceptional performance, so that's the external field.  Temperature relates to how willing people are to switch between standing and sitting.

I wrote up a version of the algorithm in Python, which you can try for yourself – I added a bunch of comments, so I hope it's not too opaque.  Here are the results for a performance not quite up to snuff:

And one that got the pixel people a bit more excited:

The only difference in conditions for these was the "performance quality" (external field): 0.1 for the first one and 0.5 for the second.  If you come up with any interesting settings yourself, be sure to leave a comment!

It's Not the Fall That Kills You

This week, I'd like to get back to one of the mainstays of this blog: Looking at physics in popular media.  A recent favorite movie of mine is the 2015 film Tomorrowland, about a secret pocket dimension where the world's greatest scientists are allowed to do their work in peace.  The central message is that an important part of science is hope for the future, that all is not lost.  Helpful thing to remember after my midweek post.

The opening scene is George Clooney's character, Frank, as a young boy inventing a jet pack.  On his first trip to Tomorrowland, he falls off a ledge, but is able to catch his jet pack, put it on, and stop just before hitting the ground.  Every time I've watched this scene, I've been skeptical that he could survive such a rapid acceleration.

I looked around for human g-force limits, and one of the largest values was for a rocket sled, which had an acceleration of 46.2 g.  A rocket sled sits on a rail, and a rocket at the back propels it (image from Wikipedia):

This is a little different from a jet pack, since it has a seat that can cushion some of the acceleration, so I'm not sure how accurate a limit that is.

In the movie, Frank falls for about 65 seconds before turning on the jet pack.  On Earth, it only takes a person 15 seconds to reach 99% of their terminal velocity, 54 m/s.  Tomorrowland looks to have similar gravity and atmosphere, so Frank is certainly going this fast by then.  When he fires the jet pack, he comes to a stop in about 2 seconds, which works out to 2.8 g, nowhere near fatal!

In case you're wondering what would happen without air-resistance and terminal velocity, even that isn't necessarily fatal: 32.5 g wouldn't be fun, but he would probably get away with only some dislocated shoulders.

A Personal Note

A few days ago, a family friend asked me to make a video for her class of 6th graders explaining what it means to be a physics graduate student, why I chose it, and what I like about it.  That, coupled with the recent election, got me thinking about how I got into physics in the first place.  I took my first physics class in 10th grade, with a wonderful teacher named Ben Bakker.  Something I immediately noticed was that he always seemed to be in awe of the universe.  He would write an equation on the board, step back, and say, "Whew, isn't that beautiful?"

The moment I remember when I really got a clear sense of that beauty was during a lab on projectile motion.  We were given spring-loaded cannons that shot ping-pong balls, and allowed to fire a few times with it pointed horizontally.  Based on the muzzle velocity we measured from that, we determined the angle to fire the ball through a small hoop a distance away.  The ball sailed through the center on the first try.  Even with all the approximations (no air-resistance, limited distance/angle accuracy) the science worked.  To me, that was beautiful, and I've seen so much more since.

Earlier this year, Neil deGrasse Tyson came to speak at the University of Michigan.  His talk was on the value of science to a society.  One of his big examples was that fact that most stars were given Arabic names, because Islamic countries were leaders in science during the middle ages.  In the modern era though, science has been devalued, resulting in the region's decline.

Last night, Tyson was on The Late Show, and encouraged everyone to make an effort to learn and teach for the next four years:

As a scientist, I find the skepticism surrounding issues like climate change deeply troubling.  Even though I have never studied climate science myself, I understand the scientific method, and the vast majority of climate scientists have agreed that there is a causal link between CO2 levels and global temperature.

I hope by writing this blog I can get just a few more people interested in science, and help them understand its value and its beauty.

The Living Daylights

Last weekend, I was doing some research work on a computing cluster in Germany, and the time stamps suddenly jumped by an hour – Turns out the EU changes from daylight saving time on the last Sunday of October, rather than the first Sunday of November.  I had been under the impression (along with many others) that DST was introduced for the benefit of farmers, but they actually lobbied against it.  The original intent was to give more daylight after the work day, to encourage people to shop.  I thought I'd take a look at how this idea lines up with the sunrise/set times from the US Naval Observatory.  Here are the times using standard time all year:

The black lines show my usual wake/sleep times.  Now if we introduce DST:

By using DST, I'm awake during more of the daylight.  This is the rationale behind one of the other arguments for changing clocks – energy savings by reducing light/heat use.  It's easier to see if we look at the overlap between waking and daylight in the two systems:

The idea that DST saves energy is disputed though, and a number of studies have not shown a conclusive benefit, so it's likely a small effect if any.  Seems likely to stay though, since getting rid of DST would take more effort than it costs to keep around.  I'll just have to keep a close eye on my foreign time stamps.

Spinning My Wheels

This week I changed the toilet paper in my bathroom, and it got me thinking about the rotational properties of a roll – When you try to spin something, you have to fight against rotational inertia, which depends both on mass, and how far from the center it's distributed.  That's why figure skaters speed up their spin when they pull their arms in:

What made me curious is that as the roll turns, the radius decreases, which lowers both the inertia and the torque that spins it.  Do these balance out?  I figured I'd try modelling what happens if you drop a roll while holding one end.

Since we're only interested in the roll rotating in the usual way, we can consider things in 2D:

We can find the rotational inertia by thinking of the roll as a disk with radius r(t), minus a smaller disk of radius r_i:

where ρ is the mass-density of the roll.  Now we can set up a bunch of other equations that we need.  By holding on to the end, we're applying a torque:

where α is the angular acceleration of the roll.  If we suppose each sheet has thickness T, we can relate the radius to the angle the roll has turned through:

Finally, we can integrate to connect the angle to the distance the roll has dropped:

Combining the torque and radius equations gives a differential equation:

This is fairly simple to solve by integration:

but I'm having trouble getting a plot.  I guess this is another week with complex equations leading to unsatisfying results.  Thanks for sticking through :)

MeteoRing

Shortly after I mentioned my girlfriend Marika, I'm delighted to announce we got engaged!  A few days later, we went shopping for my ring, which arrived earlier this week.  The metal used in the ring comes from a meteorite that hit the Earth during prehistoric times, and scattered over 8,000 square miles in the region of Africa that gives the meteorite its name: Gibeon, Namibia.

The unique design on the surface of the ring is called a Widmanstätten pattern, and is evidence of its extraterrestrial origins.  The pattern forms when a mixture of nickel and iron cool over a long period of time, on the order of 10 million years.  Such slow cooling isn't possible on Earth, where the rest of the planet can serve as a heat-sink.

The name "Widmanstätten" comes from Count Alois von Beckh Widmanstätten in 1808, but the first published study was actually from G. Thomson in 1804.  There's quite a story behind why he was not acknowledged (from Wikipedia):

Civil wars and political instability in southern Italy made it difficult for Thomson to maintain contact with his colleagues in England. This was demonstrated in his loss of important correspondence when its carrier was murdered. As a result, in 1804, his findings were only published in French in the Bibliothèque Britannique. At the beginning of 1806, Napoleon invaded the Kingdom of Naples and Thomson was forced to flee to Sicily and in November of that year, he died in Palermo at the age of 46.

It seems like a perfect choice for an astrophysicist, and a wonderful start to the next chapter of our relationship.  Thanks Marika!

Not Quite "Beam Me Up"

Last month, a group from Calgary announced they had broken the record for quantum teleportation over fiber-optics, so I thought I might talk a bit about the idea of quantum entanglement.

One of the main concepts behind quantum mechanics is that every particle has a set of states that can be measured.  It's possible for a particle to be in a mixture of several states, but once you measure a property, it "collapses" into a single state.  This idea is usually introduced with the property of spin, which you can think of as an arrow pointing out of the particle.  We can detect this spin with something called a Stern-Gerlach apparatus, which you can think of as a box with one input, and two outputs:

 

The two outputs tell you whether the spin is aligned with the angle of the box (+) or opposite the box (–).  If the particle goes in sideways, as shown above, then there is a 50% chance of it coming out each port.  However, when it comes out, it will have changed its spin to straight up or down, and all information on its previous sideways state will be lost.  In quantum mechanics, the initial sideways state is described as a superposition of the + and – states of the box: The particle is literally in both states at the same time.

Entanglement refers to two (or more) particles whose states depend on each other.  In the framework we've been discussing, we could imagine a device that puts out pairs of particles that have opposite spin.  Once we measure the spin of one, we immediately know what the spin of the other is.  This instant change of state for the unmeasured particle is called quantum teleportation.  If we put one of the particles in the box above, and it came out +, we would immediately know that the other particle was aligned opposite the box, no matter how far we had separated the two particles before making the measurement.

The idea of the new state being transmitted instantly over a great distance might worry you, since that would appear to be information traveling faster than light, which Einstein showed was impossible.  However, the state the two particles end up in is still random, even if they're guaranteed to be opposite each other.  The only way to observe this correlation is by comparing the spins conventionally at sub-light speeds.

Richard Feynman once said, "If you think you understand quantum mechanics, you don't understand quantum mechanics," but I hope I've given some insight into one of the frontiers of modern physics.  As always, questions are welcome!

Let Them Heat Cake

[Programming Note: I've noticed looking through older posts that the equations have picked up extra + signs, due to my impolite hotlinking.  I plan to go through and fix those when I have a chance, but until then, don't take them at face-value.]

Another great question from a reader, this time my own mother, Sally: I’m making a carrot cheesecake for Steve’s birthday. The cheesecake layer is supposed to go into an 9” springform pan but I only have 8”[...] I assumed I’d just make the cheesecake layer thicker, but how to determine baking time? The volume is the same but the height is 25% greater. Thicker tends to mean longer baking. But how do i determine that? What role does oven temp play? What role does moisture level of cheesecake play?

I've talked about heat transfer before, in this post about making granita, but I'd like to take a different approach this time, using the more general heat equation:

This says that the rate of temperature change at a point in the cake is proportional to the variation in temperature nearby, and the thermal diffusivity, α.  This quantity depends on the substance we're interested in, but I don't think it's been tabulated for cheesecake batter, so we'll assume it's about the same as water, 0.143 × 10⁻⁶ m²/s [this is the role the moisture level plays].

Technically, this is a 3-dimensional problem, but thanks to the cylindrical symmetry of the cake, we can just consider a 2d cross-section through the center.  We can assume the outer surface of the cake is fixed at the oven temperature, 325°F.  Then we can use a numerical solver to find the temperature throughout the cake over time.  As it happens, I wrote a solver for this equation a few years ago for a Computational Physics class.  After a few adjustments, it was ready to go: bake.py

First, we need to find the internal temperature that the cake reaches after the prescribed 45 minutes of baking.

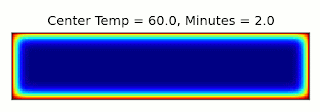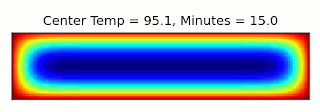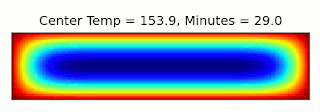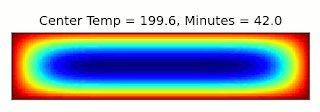
The final temperature after 45 minutes is 206°F.  This is a little close to the boiling point of water, 212°F, where I expect things to get a bit off from the approximations I'm making, but we'll go with it.  Then we can start again with a narrower cake of the same volume, and find how long it takes to get to that temperature.

The final time is about 70 minutes, which isn't completely unreasonable, but I take no responsibility for any charred cakes this calculation results in.  Thanks for a great question, Sally!

[Edit: One first posting, I mistakenly used 8/9" as radii, rather than diameter.  It doesn't actually make a difference in the final results, since the thickness dominates.]

Precipitous Power

We've been getting a lot of rain here in Ann Arbor the last few days, and it reminded me of a question I've often wondered after being hit in the head by a particularly large drop: How much power could you generate from rainfall?

The kinetic energy of each drop is

where m is the mass and v is the velocity when it hits the ground.  Clearly we need some statistics about raindrop size; a quick search turned up this paper, from a collaboration between Brookhaven National Laboratory and a group of Chinese institutions.  The important info we need is in Fig. 5:

The y-axis, volume-mean diameter, refers to the diameter of a perfect sphere with the same volume/surface area ratio as the drop.  Coincidentally, this is also known as the Sauter mean diameter.  Unfortunately, the paper doesn't provide a fitting function for these curves, but we can take a guess, estimating values from pixels:

x	y
1.149825784	0.8509578544
3.066202091	0.9689655172
4.982578397	1.078544061
7.857142857	1.137547893
13.79790941	1.230268199
27.21254355	1.348275862
48.67595819	1.390421456

I chose the convective points for their larger range of rates.  Based on those, I asked R for a log fit and got this, with an R-squared of 0.992:

The relationship between mass and diameter is

where ρ is the density of water.  We still need to find v, but if we assume the drops hit terminal velocity for a sphere, we can use eqn 3 from this paper:

where g is the acceleration due to gravity, and I've substituted in the drag coefficient for a sphere.  Putting these together, the energy becomes

This is the energy of each drop, so we need to multiply by drops per time to get power.  The drops per time is related to the rain rate from above:

where A is the collection area.  Putting everything together, we have

If we plug in values, this comes to

At the highest rain rate from the paper, this comes to 0.081776 Watts per square meter, while solar cells can put out a couple hundred Watts per square meter, so maybe not the solution to our energy problems!

This one wound up being a bit dense, sorry.  I'll try to pick a more accessible topic next time!

Lunar Loop-the-Loop

This question comes from my girlfriend Marika's grandfather, who studied physics in his youth: What shape does the moon trace as the Earth orbits the Sun?

The idea is, the Moon is going in (approximately) a circle around the Earth, while the Earth is going in (approximately) a circle around the Sun.  This is a surprisingly simple situation to model using a parametric equation:

The 'R's are the radii of the Earth's and Moon's orbits, and the 'T's are the time each takes to complete a circuit.  This type of curve is called an epitrochoid, and can take on some interesting shapes:

 

The general form for this type of curve is

We can relate this to the earlier equations with

The site I linked above states that there will be loops if b > a/c, so let's plug in some values (from Wikipedia):

Nowhere close, a result borne out by the plot:

If you look carefully, you can see that's not quite circular, but it's awfully close.  Thanks for a great question, George!

[Edit: If you're curious about the b > a/c condition, you can think of it this way – There will be a loop anytime the Moon is moving backwards faster than the Earth is moving forwards.  The speed of each is given by 2πR/T, and if you plug in the R and T relations for a, b, and c, you'll see that's exactly what b > a/c means.]

I Can RELATE!

After an exceptionally long break, I'm back again!  It turned out the combination of coursework and cancer recovery were too much to handle on their own, let alone continuing with this blog.  I took a break from my program for a year and a half to work for a private company, then came back and finished up classes and exams.  I'm now doing full-time research with my advisor Keith Riles, studying gravitational waves using LIGO.  This summer, I participated in a seminar called RELATE – Researchers Expanding Lay-Audience Teaching and Engagement.  The program culminated in recording a short video summarizing our research, which I decided would be a great way to bring back this blog.  Take a look:

As always, questions are welcome!  I'm hoping to be back here about once a week; in the years I've been away, I've kept a list of potential topics, but I can always use more suggestions.