This past week, my friend Kevin Labe let me know his group was going to be making an announcement of some exciting results from their experiment, called "Muon g-2". The experiment relates to the predictions made by the Standard Model about how charged particles behave in a magnetic field. Before we get to the results of the experiment, let's talk a bit about what is expected.
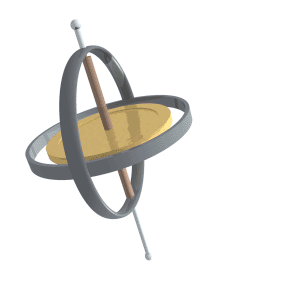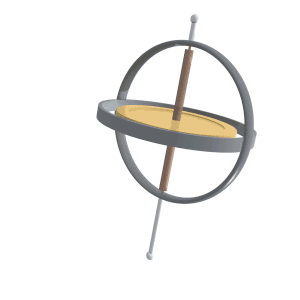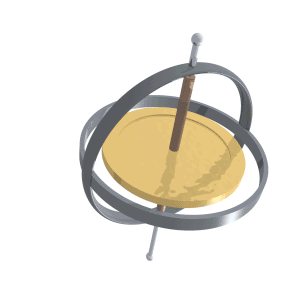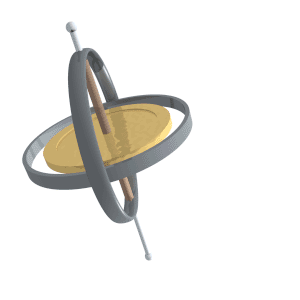
Subatomic particles have a property called spin, which is related to their angular momentum. Since we're talking about a scale where quantum mechanics applies, it's not completely analogous, but you can imagine a gyroscope:
If the particle also has an electric charge, that spinning results in magnetism – Charge moving in a circle generates a magnetic field, like the coils in an electromagnet. If we put that particle in a magnetic field, it will precess (wobble), just like the gyroscope above precesses in the gravitational field. The strength of the particle's magnetism is called its magnetic moment, and it determines how quickly the particle precesses.
Measuring a particle's magnetic moment is exactly how an MRI scanner works, though in that case we measure the moment to identify the particle, while here we are specifically measuring muons. The magnetic moment is theoretically given by
where
e is the charge of the particle,
m its mass, and
S its spin. The bit in question is the factor
g. At first, theoretical physicists thought
g was exactly 2, but after further calculations found that it was slightly more than 2, leading to discussions of the
anomalous magnetic moment and experiments measuring
g minus 2.
The reason that
g is not exactly 2 is because on a quantum scale, when particles interact they can exchange "virtual particles" which appear and disappear in the process of the interaction. These are usually represented with
Feynman diagrams:
You read these diagrams from left to right; in this example an electron and a positron (which travels opposite the arrow's direction, since it's an anti-particle) combine to make a virtual photon (blue). This photon then turns into a quark and anti-quark, which releases a gluon (green). Often the same initial and final particles will have many different paths they can follow – different types of virtual particles forming in the middle. Each of these paths will change the properties of the interaction.
Now back to the case at hand: Theorists have tried to account for all the possible virtual particles, and come up with a value for g that falls just above 2 (I won't try to express the exact value). Prior to the experiment at Fermilab, the only group that had tried to measure g experimentally was at Brookhaven National Lab. However, the result they found was outside the range predicted by theory. Their error though was not quite at the 5σ, or 5 standard deviations, level required to claim a discovery.
Fermilab's big announcement was that not only had they improved on the BNL error with a significance of 4.2σ, but their result was consistent with the previous measurement. If correct, this implies there are virtual particle interactions not accounted for by the Standard Model, i.e. new particles. Congratulations, Kevin, on being a part of this exciting work! Perhaps the explanation will be the formation of a virtual Labeton...


No comments:
Post a Comment