Birthday Bifurcation

Today is my 32nd birthday, and since 32 is 2^5, or 0b100000 in binary, I've got binary trees on my mind! A binary tree is a way to order data, and assign labels to groups. The typical structure is that you have a collection of connected nodes. Each node branches to two nodes below it, usually representing things less than, and greater than the head node. In the case of my life, we can consider things before and after I was 16, then things before and after I was 8, and so on, splitting in half each time. For example, here are all the places I've lived:

Because there's a green dot at the 1/2 level, we can tell I lived at least half my life in Ashfield, and the red dot at 1/8 means it took more than 12% of my life to get my PhD!

I'm now 10 years out from my cancer treatment, and so life sans brain tumor is growing in size:

On a lighter note, I'm now 4 years into marriage with a wonderful woman, without whom I could not have made it this far:

Marika and I have only been together for 1/8 of my life so far, but she is a bigger part of my life than I can ever express. I look forward to many more birthdays together to come!

Stochastic & Fantastic

As I've mentioned before, I keep a list of potential topics that I choose from now and then, and this week I thought I'd look back at an article that caught my interest 2 years ago. Scientific American had a story about a beetle that looked for recently-burned forests using a process called stochastic resonance. The beetles use this process to sense heat from great distances, when normally those heat signals would fall below the background levels. Paradoxically, they do this by adding more noise to the signal. I was curious if I could model this type of effect, to get a better feel for how it works.

In its simplest form, we have 3 parameters for this system: The signal strength, the amount of noise added to that, and the threshold for detection. The principle is that even if the signal is smaller than the noise, we still have signal + noise > noise. That means if we can pick our threshold so that noise < threshold < noise + signal, we'll be able to pick up the signal.

Following an example used in the Wikipedia article above, I decided to use a black & white image as the target signal. I settled on one of the more iconic photos of a certain physicist. Below, you'll find the 3 controls I described. Try turning down the overall signal, then adjust the noise and threshold to pick out different features.

Deluxe Model

This past week, my friend Kevin Labe let me know his group was going to be making an announcement of some exciting results from their experiment, called "Muon g-2". The experiment relates to the predictions made by the Standard Model about how charged particles behave in a magnetic field. Before we get to the results of the experiment, let's talk a bit about what is expected.

Subatomic particles have a property called spin, which is related to their angular momentum. Since we're talking about a scale where quantum mechanics applies, it's not completely analogous, but you can imagine a gyroscope:

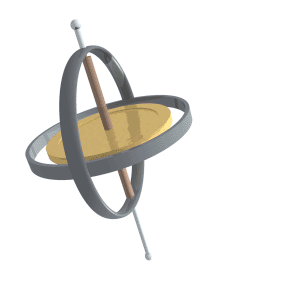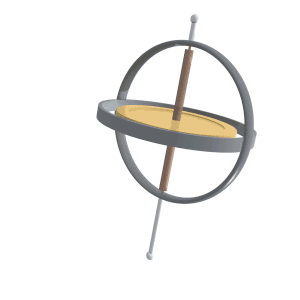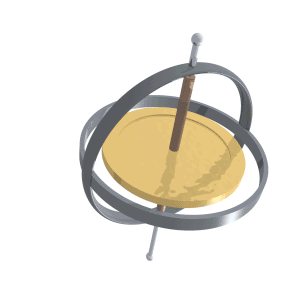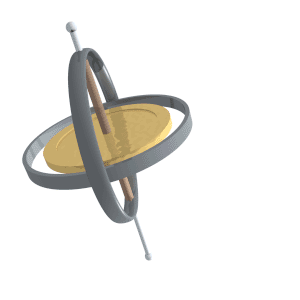Wikipedia

If the particle also has an electric charge, that spinning results in magnetism – Charge moving in a circle generates a magnetic field, like the coils in an electromagnet. If we put that particle in a magnetic field, it will precess (wobble), just like the gyroscope above precesses in the gravitational field. The strength of the particle's magnetism is called its magnetic moment, and it determines how quickly the particle precesses.

Measuring a particle's magnetic moment is exactly how an MRI scanner works, though in that case we measure the moment to identify the particle, while here we are specifically measuring muons. The magnetic moment is theoretically given by

where e is the charge of the particle, m its mass, and S its spin. The bit in question is the factor g. At first, theoretical physicists thought g was exactly 2, but after further calculations found that it was slightly more than 2, leading to discussions of the anomalous magnetic moment and experiments measuring g minus 2.

The reason that g is not exactly 2 is because on a quantum scale, when particles interact they can exchange "virtual particles" which appear and disappear in the process of the interaction. These are usually represented with Feynman diagrams:

Wikipedia

You read these diagrams from left to right; in this example an electron and a positron (which travels opposite the arrow's direction, since it's an anti-particle) combine to make a virtual photon (blue). This photon then turns into a quark and anti-quark, which releases a gluon (green). Often the same initial and final particles will have many different paths they can follow – different types of virtual particles forming in the middle. Each of these paths will change the properties of the interaction.

Now back to the case at hand: Theorists have tried to account for all the possible virtual particles, and come up with a value for g that falls just above 2 (I won't try to express the exact value). Prior to the experiment at Fermilab, the only group that had tried to measure g experimentally was at Brookhaven National Lab. However, the result they found was outside the range predicted by theory. Their error though was not quite at the 5σ, or 5 standard deviations, level required to claim a discovery.

Fermilab's big announcement was that not only had they improved on the BNL error with a significance of 4.2σ, but their result was consistent with the previous measurement. If correct, this implies there are virtual particle interactions not accounted for by the Standard Model, i.e. new particles. Congratulations, Kevin, on being a part of this exciting work! Perhaps the explanation will be the formation of a virtual Labeton...