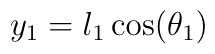Interference Inference

Last night I slept at my parents' new condo for the first time.  It's a nice place, but it's next to a couple busy streets, so there's a lot of car noise.  It got me thinking about active noise canceling, where a device picks up the background noise and replays it delayed by just the right amount that the two waves cancel.  Here's a quick example:

If the black curve is the original, the noise canceling device would produce the red curve.  Adding them together gives zero, no sound.

Things are a little more complicated in three dimensions, but it's still possible.  Let's say I want to cancel the noise from the street while I'm in bed.  I can put a speaker in a specific spot that replays the street noise shifted slightly.  The animation below shows the contours of the total of the two sound waves.  I'm at the blue dot.  Ideally, a silent spot would be a place that no contour lines cross, so I didn't get this tweaked perfectly, but you can see that the blue dot will be a lot quieter than other places.

 

One potential problem with this idea is the frequency of car noise.  It's around 1000 Hz, and a quick calculation shows it has a wavelength of about 13 inches.  That means that if you set things up to cancel at one ear, half a wavelength away at your other ear, the waves could add, making the noise twice as loud.  I might be better off with earplugs...

Here Comes the Sun

While I've been home, I've been looking at Steve's weather station.  I hadn't noticed before, but in addition to temperature, humidity, and all the standard weather statistics, it also measures solar radiation and UV index.  Here are the plots of those two for the past 24 hours:

Today's been pretty cloudy, so things aren't that exciting, but there was a nice peak at 2:00 yesterday.  UV Index is a sort of strange parameter.  It's designed to be a simple indication of how damaging the sunlight can be to your skin.  Originally, it was meant to be on a scale of 0-10, with 5 and up indicating a risk of damage without sunscreen.  However, with the depletion of the ozone layer, the index can now rise above 10 in some places.

Looking at these plots together, I started wondering how much of the total solar radiation was UV light.  Unfortunately, the UV index is calculated by weighting the UV radiation with a function called the McKinlay-Diffey Erythema action spectrum.  The function varies according to how dangerous specific wavelengths are to your skin, but it never goes above 1, so we can at least get a minimum measure of the UV radiation.

At 2:00 yesterday, the UV index was about 4.25.  To convert this into radiation, we divide by 40, giving 106 milliwatts/meter^2.  The total solar radiation at that time was about 700 watts/meter^2, so the UV light made up a minimum of 0.01% of the radiation reaching Earth.  Not very much, but it goes a long way.

The Atomic Terrier

The differential equations from yesterday continue to stump me, so they may have to wait until I'm back at my main computer, where I can try some more advanced calculations.  This ancient laptop is just too clunky...

In the meantime, I thought I'd share something I noticed about our dog Darcy the other day.

If you look closely at her license...

...you'll see she's number 238.  Uranium-238 is the most common isotope found in nature, and is used in breeder reactors to make Plutonium.  It is also used in nuclear weapons to increase the yield (that is, make it more powerful).  An appropriate number for so volatile a dog...

Wag the Dog, Part 1

I'm at home in Ashfield for the week, which means I get to spend time with our two dogs, Ida, a yellow lab, and Darcy, a Boston terrier.  Both dogs have an interesting habit of wagging their tails so violently that their whole body begins to wag too.  I put together a little illustrative animation:

The first thing that came to mind when I saw this was coupled oscillation, when two oscillators are connected, and the movement of one causes the other to begin moving as well.

When dealing with coupled oscillators, it's often useful to find their normal modes.  First though, we'll need to come up with some equations that govern this motion.  I'm thinking the best model is a sort of angular spring – the more a joint bends, the harder it tries to return to straight.  In this case, the equation for the joints would be

where τ is the torque, θ the angle the joint is bent, and k the spring constant.  We can turn the torque into a more useful measure of the joints' movement by using the moment of inertia of a rod, I.

where α is the angular acceleration, m the mass, and l the length of the segment of body.  In order to use the standard methods for coupled oscillators, we'll need to put this in Cartesian (x and y) coordinates.  Since the upper joint is fixed, we'll take as our points of interest the lower joint and the tip of the tail.  If we know where these two points are, we can find the positions of everything else.  For the lower joint, we have

and for the tip of the tail

so solving for the θs,

We also need the second derivatives of each of these. Starting with the first derivatives,

Then the second derivatives are

Whew, things are looking pretty ugly.  I'm going to stop here for now and pick this up again tomorrow.  Sorry to leave you hanging...

Keep on Truckin' Correction

When I woke up this morning, I suddenly realized I had made a mistake in yesterday's post – I was looking for a maximum in the E/s function, when really I wanted a minimum. It turns out that for a > 2, there is such a minimum. We can find it by taking the derivative of Q with respect to s.

Then to find the minimum, we set this to zero and solve for s.

Plotting this, we see that as a increases, we approach the limiting case of stretching your legs as far as they can go, but for a near 2, you're better off with smaller steps.

Keep on Truckin'

Apologies to anyone frantically refreshing the page looking for a new post; this was my birthday weekend, and my girlfriend, Jen, came out for a visit.

Due to my chemotherapy treatment, I've been spending a lot of time in bed, so Nurse Steve has been diligent in taking me on forced marches through Boston to keep me in shape.  The wheelchair calculations I did earlier got me thinking about the mechanics of walking.  Specifically, it occurred to me that though a longer stride allows you to cover more ground, it causes your body to move up and down more – as your leg goes out, you drop slightly, and as you bring your back leg forward, you return to your full height.

Let's say your legs are a length l and you take strides of length s:

Then your lowest height is

and with every step your change in height is

so every time you lift yourself up, you expend an energy of

Clearly if you wanted to expend the least energy, you'd just take infinitesimal steps, but you'd never get anywhere, so let's define a step quality, Q, as the energy over the stride to some power a (a scaling factor):

As you can see from the plot of this function, the results are a little disappointing. Depending on how much you value stride over energy, it's either best to stretch all the way to the ground, or to take the tiniest steps you can. Oh well, you can't get interesting results every time...

[This conclusion has an error.  You can see my correction here.]

Physics Fought the Law, and Physics Won

Earlier today, I spotted this news article about a man who successfully disputed a speeding ticket given by an automated camera.  He superimposed the pair of timestamped pictures and showed that his speed could not have been the 50 mph that the ticket claimed.  The camera company countered that the speed is measured 50 feet before the photos are taken, so he may have slowed down.  This made me wonder exactly how fast he would have to be braking for such a theory to work.

Suppose he was going 50 mph when his speed was measured, then he braked to reach 35 mph (the speed limit) 50 feet later.  His acceleration is given by

Where vf and vi are the final and initial velocities, and t is the time spent decelerating.  Of course, we only know x, the distance he traveled, not t, so we have to find it using

Substituting into the original equation gives

or resolving for a,

Plugging in the values we have, the car would be decelerating at 15% the force of gravity.  According to Wikipedia, some roller coasters get up to 300% the force of gravity, so maybe the situation is more plausible than I first thought.  Whether the man was speeding or not, I commend him for using physics and the information available to him to make a convincing argument for his innocence.

Fit vs. Fat

Last Monday was the Boston marathon.  Steve and I walked out to see a section of the race close to the finish line.  The marathon includes a wheelchair group, a few of whom we saw go by.  I was surprised by the variety in wheelchair designs, since it seemed like this could have a big impact on a racer's performance.  As I started thinking about it, it occurred to me that even identical wheelchairs could perform differently due to their rider's weight.  Compared to running, wheelchairs preserve momentum much more efficiently, so even though it would be harder for a heavy person to get up to speed, they would stay there much more easily.

Consider a pair of racers, identical except for their weight.  They each have an amount of energy, E, to expend during the race.  They will each race by accelerating to some top speed, vr, where r is 1 for racer 1 and 2 for racer 2.  After reaching top speed, they will remain at that speed for the remainder of the race.  The racers' net force will be given by

where mr is the mass of, ar the acceleration of, and Fr the force exerted by racer r, and Crr is the rolling resistance coefficient.  The energy the racer needs to use then is

With our assumptions about acceleration, we can simplify this to

where xar is the distance spent accelerating and xsr is the distance spent stable of racer r.  However, we're more interested in the time spent accelerating and stable.  The conversion for the stable period is easy,

The conversion for the accelerating part requires a bit of integration, but is pretty standard

So then we have

We want to minimize the racers' total time by varying their top speed, so we differentiate

Since we want

we have

We are still left with a derivative of tsr, but we can set this to zero on the grounds that tsr should be maximized.  Solving for vr gives then

The derivative of E is the same for both racers, so we can just treat it as a constant.  Plugging vr into our equation for E gives

or simplifying,

We're more interested in total time, so we substitute in

Solving for tr then

Clearly, this will be minimized when

Solving for mr,

This is the ideal, and also the maximum, mass under these assumptions.  It does strike me as a little strange that a larger mass would cause tr to become imaginary, but I suspect this is due to mr and E being related.

Also interesting is the implication that, with the ideal mass, the best strategy is actually to accelerate during the entire race, only reaching half maximum speed by the end.  I'm concerned there's a mistake somewhere in here, leading to these strange results, but I don't see it.  Let me know if you notice something amiss.

Super collide 'er? I just met 'er!

Credit goes to Humorbot 5.0 of Futurama for the title.

I know I said this was going to be about everyday stuff, but in a few months, this will be everyday for me.  Starting in June, I'll be getting proton radiation therapy at Massachusetts General Hospital as part of my cancer treatment.  As a physicist, I'm excited by the idea of having protons fired into my brain, and I decided to look into some of the details of the cyclotron that will be providing the protons.

For those unfamiliar with the term, a cyclotron is similar to a supercollider, but only accelerates particles, rather than smashing them into each other.  It is built as a ring (hence 'cyclo-') with a series of magnets around the circumference.  The magnets gradually accelerate the particles as they circle the ring, until they get to the desired speed.  Generally, the particle speed is given in terms of how much energy the particle has.  The MGH cyclotron gets the protons up to 235 MeV, where MeV is mega electron volts, the energy contained in a million electrons after accelerating through one volt of potential difference.  We can convert this to a velocity using the relativistic kinetic energy equation:

where v is the velocity as a fraction of the speed of light.  Solving for v and plugging in values gives the velocity of the protons as 60% light speed.

As far as relativistic particles go, this isn't stunningly fast, but it's certainly fast enough to get some neat effects.  If there were a clock on the protons that we could read, we would see 4 seconds tick by for every 5 seconds that passed on our clocks.  According to the specs of the MGH cyclotron, it takes 800 turns around the loop to get up to the correct speed.  I couldn't find exactly how big the MGH ring is, but a similar cyclotron has a diameter of 6.6 meters.  Some quick algebra gives the total distance traveled as 16.6 kilometers.  According to us, that trip will take 92.2 microseconds, but if you were riding on the proton, it would only seem like 73.8 microseconds.

Another interesting property of the protons is their Bragg peak.  This is the depth at which the proton deposits the majority of its energy in the material it's traveling through (in this case, my brain).  This plot compares the Bragg peaks for protons and photons (from Wikipedia):

 Notice that the photon (used in typical radiation treatment) peaks almost immediately, and stays relatively high for a significant distance.  The proton, however, peaks sharply at a specific depth, then drops to zero almost immediately.  This is the main advantage of proton therapy over traditional radiation treatments – very little is irradiated aside from what is targeted, reducing side effects.

I hope you haven't been put off by the cancer talk – I'll start on more everyday things tomorrow.